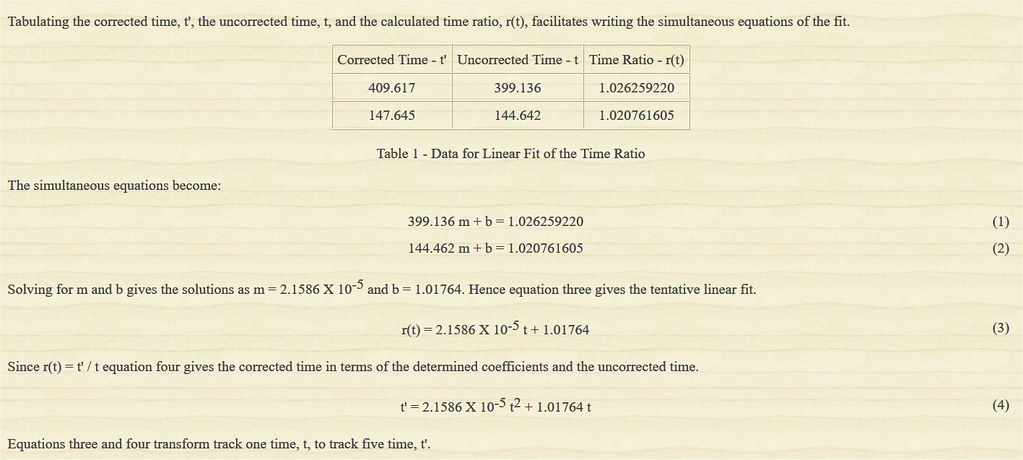
Post by Herbert Blenner on Feb 9, 2019 18:10:27 GMT -5
Thump, Thump, Thump
by Herbert Blenner | Posted June 21, 2011
Source: BB&N - 8HSCA, 73
Figure 9 illustrates the results of stopwatch timing of the Dictabelt record of the events on Channel 1. Here, the slope of the least-square error fit is 0.95, indicating that the recorder was running 5% too slow and, therefore, was compressing time slightly. * The fact that the slope does not change over the course of the entire segment shows that the recorder operated continuously.
*Frequency analysis of the power hum on the tape recording also indicated that the recorder had been about 5% slow. Since the hum could have been added when the tape was recorded from the dictabelts, this is not a reliable indication of the original recording speed.
A power line hum of sixty cycles per second is placed on a recording as a density of cycles per inch. In particular the density, d, equals the frequency of the signal, f, divided by the recording speed, r, typically express as inches per second.
d = f / r
Upon playback the machine converts the recorded density, d, to a new frequency, f ', that equals the density multiplied by the playback speed, p.
f ' = p d
Combining results gives the new frequency, f ' as the original frequency f multiplied by the playback speed divided by the recording speed.
f ' = ( p / r ) f
James Bowles slowed the playback of the Dictabelt during his taping and made the playback speed less than the recording speed. So the 60 Hz power line hum was recorded on his tape at a lower frequency. Measurements from his tape give this lowered frequency as 57. 3 Hz and the ratio of his playback to recording speeds was 57.3 / 60 or 0.955.
Source: BB&N - 8HSCA, 110
The tape-recording system was found to be about 5% slow, when the time annotations were measured with a stopwatch (see Fig. 9). Therefore, the apparent pitch of the tone would have a frequency of (1.05) (420) = 441 Hz.
The correction factor for frequencies on the Bowles tape becomes 1/0.955 or 1.047. So the true frequency of the power line hum, f, equals the 1.047 multiplied by the frequency on the Bowles tape, 57.3 Hz. As expected, (1.047) (57.3 Hz) = 60.0 Hz.
Likewise the same correction factor of 1.047 applies to the 420 Hz tone on the Bowles tape and the corrected frequency becomes (1.047) (420 Hz) or 440 Hz.
Source: BB&N - 8HSCA, 75
The time span between the onset of the first impulse pattern and the onset of the fourth impulse pattern on the Channel 1 tape is 7.9 sec. When corrected for the fact that the tape recorder was running about 5% too slowly, the real time span is 8.3 sec.
Time and frequency are inversely related. So the correction factor for time is the reciprocal of the correction factor for frequency. This relationship can be verified by considering the 57.3 cycles of power line hum on one second of time on the Bowles tape. Multiplying this time on the Bowles tape by 0.955 gives the true time and the corrected frequency on the Bowles tape is 57.3 cycle per 0.955 second or 60.0 cycle per second.
The 7.9-second span between the first and the fourth pulse patterns on the Bowles tape corresponds to (0.955) (7.9 second) or 7.54 second of real time.
These results are consistent with experience. If we slow the playing of a wave file the pitch of the sounds become lower and the playing time increases.
The correction factor for the frequency is a number greater than one while the correction factor for time is less than one. Working out the math shows that these correction factors are reciprocals.
Source: W&A - 8HSCA, 26
As was shown in the BBN analysis, the DPD recorder was running slow at the time the recording was made. Consequently, when the recording is played back at the faster, correct speed, the recorded impulse sounds will be heard closer together than they actually were at the time the recording was made. This error could be corrected by multiplying the time intervals measured on the graph by a time-correction factor. The BBN analysis showed that between 12:22 p.m. and 12:37 p.m., the average speed of the recorder was 0.95 of correct speed. The actual speed at any time during this interval could have been from 0.94 to 0.96 of true speed. Accordingly, the time-correction factor could range from 1.04 to 1.06.
The idea of a recorder running slow is mistaken. Instead any recording speed has a proper and equal playback speed. In particular James Bowles slowed the play back of the Dictabelt during his taping. So the proper correction for frequency involves multiplication by the recording speed divided by the playback speed. This factor is greater than one. Likewise multiplication of time on the Bowles tape by the playback speed divided by the recording speed gives the corrected time that is less than the time on the Bowles tape.
Source: W&A - 8HSCA, 27
Because any value between 1.03 and 1.07 was theoretically valid, it was permissible to choose the value between those limits that created the best match between the impulse and echo sequences. By fitting the DPD tape recorded impulse sequence to our predicted echo sequences, we found that a time-correction factor of 1.043 gave the best match, and we therefore used that factor.
The pulse pattern associated with the Grassy Knoll shot produced the best match to a test shot pattern when they multiplied time on the Bowles tape by a correction factor of 1.043. However, the slowed playing of the Dictabelt during recording of the Bowles tape would have expanded time. So a time compression of 1/1.043 or 0.959 should have produced the best match for a true Grassy Knoll pattern on the Bowles tape.
This contradictory situation arose because the studio that impressed the Grassy Knoll shot pattern on the Bowles tape made a mistake. This studio should have expanded time on their source recording so that the proper compression 0.959 would have produced the best match to the test shot pattern. Instead the studio compressed time on their source and caused the best match with the test shot to have occurred with a time expansion of 1.043.
Two possibilities immediately come to mind. First, amateurs made the alterations to the Bowles tape or devious professionals planted a clever trap on the Bowles tape. The failures of the Ramsey Panel and the Watson Research Center to highlight the mistaken 1.043 time-correction factor used by W&A supports the latter possibility.
by Herbert Blenner | Posted June 21, 2011
The report of BB&N provides the details to prove that a studio added the pulse pattern identified as the Grassy Knoll shot to the audio record of the Dictabelt.
Source: BB&N - 8HSCA, 73
Figure 9 illustrates the results of stopwatch timing of the Dictabelt record of the events on Channel 1. Here, the slope of the least-square error fit is 0.95, indicating that the recorder was running 5% too slow and, therefore, was compressing time slightly. * The fact that the slope does not change over the course of the entire segment shows that the recorder operated continuously.
*Frequency analysis of the power hum on the tape recording also indicated that the recorder had been about 5% slow. Since the hum could have been added when the tape was recorded from the dictabelts, this is not a reliable indication of the original recording speed.
A power line hum of sixty cycles per second is placed on a recording as a density of cycles per inch. In particular the density, d, equals the frequency of the signal, f, divided by the recording speed, r, typically express as inches per second.
d = f / r
Upon playback the machine converts the recorded density, d, to a new frequency, f ', that equals the density multiplied by the playback speed, p.
f ' = p d
Combining results gives the new frequency, f ' as the original frequency f multiplied by the playback speed divided by the recording speed.
f ' = ( p / r ) f
James Bowles slowed the playback of the Dictabelt during his taping and made the playback speed less than the recording speed. So the 60 Hz power line hum was recorded on his tape at a lower frequency. Measurements from his tape give this lowered frequency as 57. 3 Hz and the ratio of his playback to recording speeds was 57.3 / 60 or 0.955.
Source: BB&N - 8HSCA, 110
The tape-recording system was found to be about 5% slow, when the time annotations were measured with a stopwatch (see Fig. 9). Therefore, the apparent pitch of the tone would have a frequency of (1.05) (420) = 441 Hz.
The correction factor for frequencies on the Bowles tape becomes 1/0.955 or 1.047. So the true frequency of the power line hum, f, equals the 1.047 multiplied by the frequency on the Bowles tape, 57.3 Hz. As expected, (1.047) (57.3 Hz) = 60.0 Hz.
Likewise the same correction factor of 1.047 applies to the 420 Hz tone on the Bowles tape and the corrected frequency becomes (1.047) (420 Hz) or 440 Hz.
Source: BB&N - 8HSCA, 75
The time span between the onset of the first impulse pattern and the onset of the fourth impulse pattern on the Channel 1 tape is 7.9 sec. When corrected for the fact that the tape recorder was running about 5% too slowly, the real time span is 8.3 sec.
Time and frequency are inversely related. So the correction factor for time is the reciprocal of the correction factor for frequency. This relationship can be verified by considering the 57.3 cycles of power line hum on one second of time on the Bowles tape. Multiplying this time on the Bowles tape by 0.955 gives the true time and the corrected frequency on the Bowles tape is 57.3 cycle per 0.955 second or 60.0 cycle per second.
The 7.9-second span between the first and the fourth pulse patterns on the Bowles tape corresponds to (0.955) (7.9 second) or 7.54 second of real time.
These results are consistent with experience. If we slow the playing of a wave file the pitch of the sounds become lower and the playing time increases.
The correction factor for the frequency is a number greater than one while the correction factor for time is less than one. Working out the math shows that these correction factors are reciprocals.
Source: W&A - 8HSCA, 26
As was shown in the BBN analysis, the DPD recorder was running slow at the time the recording was made. Consequently, when the recording is played back at the faster, correct speed, the recorded impulse sounds will be heard closer together than they actually were at the time the recording was made. This error could be corrected by multiplying the time intervals measured on the graph by a time-correction factor. The BBN analysis showed that between 12:22 p.m. and 12:37 p.m., the average speed of the recorder was 0.95 of correct speed. The actual speed at any time during this interval could have been from 0.94 to 0.96 of true speed. Accordingly, the time-correction factor could range from 1.04 to 1.06.
The idea of a recorder running slow is mistaken. Instead any recording speed has a proper and equal playback speed. In particular James Bowles slowed the play back of the Dictabelt during his taping. So the proper correction for frequency involves multiplication by the recording speed divided by the playback speed. This factor is greater than one. Likewise multiplication of time on the Bowles tape by the playback speed divided by the recording speed gives the corrected time that is less than the time on the Bowles tape.
Source: W&A - 8HSCA, 27
Because any value between 1.03 and 1.07 was theoretically valid, it was permissible to choose the value between those limits that created the best match between the impulse and echo sequences. By fitting the DPD tape recorded impulse sequence to our predicted echo sequences, we found that a time-correction factor of 1.043 gave the best match, and we therefore used that factor.
The pulse pattern associated with the Grassy Knoll shot produced the best match to a test shot pattern when they multiplied time on the Bowles tape by a correction factor of 1.043. However, the slowed playing of the Dictabelt during recording of the Bowles tape would have expanded time. So a time compression of 1/1.043 or 0.959 should have produced the best match for a true Grassy Knoll pattern on the Bowles tape.
This contradictory situation arose because the studio that impressed the Grassy Knoll shot pattern on the Bowles tape made a mistake. This studio should have expanded time on their source recording so that the proper compression 0.959 would have produced the best match to the test shot pattern. Instead the studio compressed time on their source and caused the best match with the test shot to have occurred with a time expansion of 1.043.
Two possibilities immediately come to mind. First, amateurs made the alterations to the Bowles tape or devious professionals planted a clever trap on the Bowles tape. The failures of the Ramsey Panel and the Watson Research Center to highlight the mistaken 1.043 time-correction factor used by W&A supports the latter possibility.